ἀγορά

The Greek agorá (note that Ancient Greek very, very likely had tones) served as space to talk about the polis, the city, but hosted also various markets and other commercial spaces. The word has its etymology in the two Greek verbs ἀγοράζω, agorázō, “I shop”, and ἀγορεύω, agoreúō, “I speak in public” (source: wikipedia).

In these meetings, originally thought and motivated by Alejandro Torres, we meet at some nice place of PKU campus and discuss about anything which might be interesting. It will be related to our research, but in principle we can and should discuss without any kind of artificial boundaries anything intellectual that we find worth addressing.

If something related to our research comes out of the discussion, a new idea or concept, we summarise it here just to keep a log.

The meetings – Summary and log

24 April 2018
Lake Wèi Míng Hú
Chén Xián, Alejandro Torres, Verónica Vázquez, Pau

Two ideas, suggested by Chén Xián and Alejandro Torres

(1) Beaming effect of gravitational waves on light emitted by a quasar

Around a SMBH an accretion disc might form and trigger “quasar activity”. We expect sources of GWs to be very close to the central SMBH (mass segregation, clump formation in the disc, or a past one also might have formed stars which evolve to stellar-mass bhs – you can form stellar-mass binaries of bhs or EMRIs etc). This is a plus because the source of GWs is close to the source of light and, hence, the strain of the GW is increased, but the source of GWs could also be located far away. The most important point is that since the jet is very relativistic, the relative velocity is very large, and this would represent an ideal scenario for the beaming effect found by Alejandro. Another possibility would be two merging neutron stars, since the relative velocities are also high. However, the QSO scenario is probably easier to address. We should do a first-order estimate assuming the simplest conditions (QSO jet is a straight line etc) to see what are the maximum and minimum effects.

This is a plot made by Alejandro for the maximal effect a GW of the amplitude “h” would have on the time dilation for different velocities.

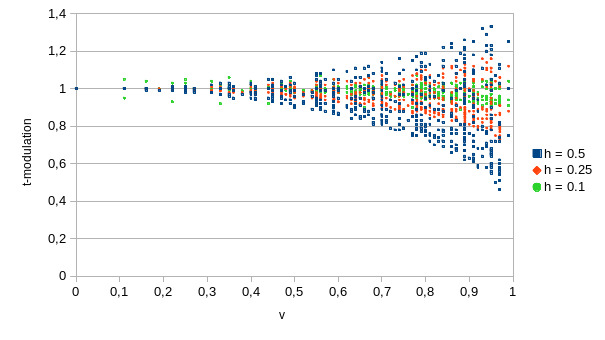
Some comments:
i) The spread for specific values of the velocity is because the effect also depends on the direction of the velocity, which is not shown here.
ii) If the GW has no influence, then the value is one. If it is bigger or smaller then one is not really important because the wave itself oscillates from -1 to +1. The
magnitude of the effect is the distance to one.
iii) This modulation is on top of the special relativistic correction. However, the beaming effect of light also depends on aberration and Doppler-shift of the frequency.
Therefore, the effect on the luminosity is (probably) smaller than the one we can see here.
iv) Of course the effect would be stronger for a bigger “h” but then we are not in the linear theory of GWs and the calculations have to be done again… maybe something we can decide to do later
v) For this plot I assume the magnitudes of the two polarizations to be the same (most likely the case for jets in Quasars). For the effect on particle accelerators on earth
this is not necessarily true, however, the effect looks quite similar for other ratios of the polarizations.

Effect of GWs on the decay of particles on Earth, more specifically on the time dilation.

Time dilation is a prediction of special relativity, which asserts that a moving “clock” seems to tick slower than a clock at rest with respect to an observer. This
prediction has been verified by various laboratory experiments which basically employs fast-moving (relativistic) particles:

https://en.wikipedia.org/wiki/Time_dilation#Experimental_testing

However, under the influence of GW, the relationship between the proper time (of the moving clock) and the coordinate time (of the clock at rest) differs from the classic
relationship predicted by special relativity, as Alejandro has discovered in his derivation of the beaming effect of GWs. Is this difference detectable in laboratories? Or it
actually already has been detected but, previously without knowing the influence of GWs, has been interpreted differently?

---

15 May 2018

KIAA and La Sapienza (Pau)
Chén Xián, Alejandro Torres, Verónica Vázquez, Pau

Two ideas suggested by Pau

Understanding the effect of time dilation

(1) EMRIs: An external observer will never see matter crossing the event horizon of a black hole. What consequences does this have for sources of GWs? Being more specific, an EMRI freezes an epsilon before the EH, and we lose the GW signal because of the huge redshift due to the acceleration of the source towards the EH. An external observer never sees the small black hole cross the EH. Assuming an event rate of 1e-5 per year, this would mean that any SMBH would have a frozen small black hole close to the EH every 100,000 years. This gives about 1e5 frozen black holes over a Hubble time being optimistic (since at redshifts higher than 1 or so we do not know how EMRIs should form in protogalaxies, and the dynamics might be completely different). However, the “surface” of the SMBH would be not spherical. Would that emit GWs? It would, probably, but redshifted, so that we do not see them. But what about the n+1 EMRI which arrives there? Is it going to evolve differently due to the “bumpyness” of the surface of the SMBH?

(2) SMBH and SMBH binaries: If we do not see matter crossing the EH, SMBH are not really black holes for external observers. Matter never reaches the EH, it should be distributed around the EH, very close to it. This matter is coming from gas inflow, typically, accreting on to the central black hole. This black hole should have formed at the very early universe. Hence, the SMBHs that we “see” nowadays are in reality smaller black holes surrounded by a huge, supermassive”crust” of baryonic matter. The SMBHs with masses of 1e6 – 1e10 Msun that we see are in reality smaller black holes surrounded by a crust of about the total of the mass, i.e. 1e6 – 1e10 Msun, because the SMBH has got its mass via gas accretion, mostly. Almost all of the mass is from the gas, the “baryonic crust”, which we never see traverse the EH.

(2.1) If we consider a single SMBH, from the point of view of electromagnetic observations, everything is of course identical, because for all pragmatic purposes we have a pure GR black hole with a mass of such and such and there is no way we’re going to be able to detect that system composed of a shell of baryonic matter surrounding a smaller-size black hole. All of the experiments thought of to detect SMBHs will not see any difference – There’s a deep potential well confined in a small volume and nothing else. The S-stars will keep their orbits, the Event Horizon Telescope will see the same “shadow” cast by the system of black hole + baryonic crust.

(2.2) If we consider two of these systems (SMBH+crust, which I will call SMC+bh, “supermassive crust and a black hole”), then there is a possibility that we can distinguish a SMC+bh from a pure GR SMBH. This is so because when the two SMC+bh objects inspiral, they will behave identically to a SMBH binary, but at some point tidal effects on the crust should become relevant, or maybe not, because the mass is distributed around the EHs at an epsilon away. In any case, when the two objects are merging, the effect of the two 10^6-10^7 Msun baryonic matter should become apparent and have a role in the trajectory/dynamics of the two objects as compared to two pure GR SMBHs in vacuum, i.e. without any baryonic crust.

There is some work in this direction:

http://adsabs.harvard.edu/abs/2009PhLB..679…88L

Although it seems that “it is well known that matter distributed around a black hole leads to strange things to happen”.

---

22 May 2018

KIAA and La Sapienza (Pau)
Chén Xián, Alejandro Torres, Verónica Vázquez, Pau

One old idea of Chen Xian and Pau.

The effect of GW sources on the light of regular stars and GAIA

The MW has probably up to millions of compact binaries, very likely WD binaries emitting monochromatically (for us, i.e. in a timescale of 5 years the source keeps its frequency basically unmodified). If a star is located close to one of these sources, the GW could deflect the light emitted by the star, and GAIA should be able to see it if the deflection angle is big enough. The questions that arise however are

-whether this angle would be big enough

-how would you be able to discern this effect? what would be the signature, if “it has been there all the time”?

-the starlight will not be affected by just one GW source, but by a myriad of them. What would be the background effect on to the light?

The advantages of this setup is that Alejandro can use his approach to estimate the angle, because his beaming effect has been worked out in the far field approximation.

---

07 August 2018

KIAA
Chén Xián, Alejandro Torres, Verónica Vázquez, Pau

A meaningful four-dimensional cross product

Imagine you have two vectors, let’s say one in x- and the other in y-direction, both of length one. If you take the cross product of these two vectors, you get another vector
in z-direction, again of length one. Now consider this three-bein moving with a velocity close to the speed of light in, e.g., x-direction. Special relativity says that the vector in x-direction is contracted (length is less than one) but the vectors in the other two directions should remain unaffected. However, if you first transform the vectors in x- and y-direction and then take the cross product, the resulting vector changes. It is still in z-direction but its length is not one. Here we seem to have a contradiction!

This means, if you first Lorentz transform two vectors and then take their cross product, you get another result then if you first take their cross product and then transform
the vectors according to Lorentz transformation. In other words, the cross product is not invariant under Lorentz transformations. In contrast, the scalar product is invariant under Lorentz transformations. However, this statement is only true for the four dimensional scalar product. For the three-dimensional scalar product you have a similar problem as for the (three-dimensional) cross product. This is one first hint to understand the contradiction: using the cross product we only consider a three dimensional subspace of “real” space-time, and, hence we are missing some part which could resolve the contradiction.

Some consequential questions are: Which operation, corresponding to the cross product, is Lorentz invariant? Or in other words, is there a meaningful four-dimensional cross product? What does this means for quantities defined using the cross product, e.g., angular momentum? And last but not least, what does this non-invariance mean physically? For example, what means this for the angular momentum in a system moving close to the speed of light?

---

11 Sep 2018

KIAA, Berlin, Thessaloniki
Chén Xián, Alejandro Torres, Stamatis, Angela, Pau

Moaning Black Hole model

Perturb the black hole from the inside and have a perturbed event horizon, this even horizon will generate quasi-normal like gw
candidates for perturbares are BNS systems falling into the SMBH, since the tidal seperation radius lies within the EH of the SMBH. The main idea we had is that since we are talking about spacetime itself a pertubation of it could travel in the opposite direction of the singularity, the physics of GW is different than light( which is a wave but the photon is the particle that “carries” this field ). Since there is no particle for gravitational waves we dont think that it has to travel only in the singularity direction. If we can somehow pertubate the EH, we can get maybe some frequencies like the quasi-normal modes.
Kokkotas & Stergioulas: It is possible (?!)

Higgs as a Dark Matter explanation

Place: Placa del Sol-Barcelona while drinking GOOD Sangria
Xian Chen, Alejandro Torres and Lazy Greek Guy, later Pau and Angela during skype telecon
The higgs boson gives mass to the particles -> the higgs field is not homogeneous -> Inhomogeneities should give different mass to the same particles eg protons -> this ??M maybe could explain the dark matter
Proposed experiment by Xian Chen: The observed spectra should be the same but the velosities in Binary systems or Globular clusters should be different. So we can find as much as identical we can in different dark matter distribution places in the galaxy and see if there is any difference in the velocities.
-What are the consequences of heavier particles?
-Is our idea compatible with stellar evolution theory?
-Is is compatible with galaxy evolution theory? ( dark matter forms gravity wells so that baryonic matter can fall in )
Last: We need to come up at some point with a theory to explain the inhomogeneity of the higgs field. Alejandro proposed a coupling with gravity.
Latest update: The mass is given at the big bang stage of the universe, The temperature is affecting the mass that is given by the breaking of the symmetry of the higgs field. I am somehow pesimistic that we will get something from the theoretical point of view. We can check the dispersion velocities of Globular Clusters and see if we can verify that there is any ??M, there is no current theory to explain any link of ???? inside the clusters with dark matter. This result on its own is exciting. But I dont know if the theory holds from the classical QFT side.

More notes:

i) The Higgs mechanism gives mass to all elemental particles (quarks, leptons…) and the mass they get in flat space-time (FST) is always proportional to a value v, which is not fixed in the theory but was measured.

ii) However, v can be calculated (in FST) as v = |\mu|/(\lambda^(1/2)), where \mu^2 and \lambda are two positive constants in the Lagrangian. This result can be found when calculating the vacuum expectation value (VEV) using a special gauge, which always can be found.

iii) In curved space-time (CST) the whole Lagrangian is multiplied by a factor G:=(-g)^(1/2)/c, where g is the determinant of the metric tensor. This product transforms the two constants \mu^2 and \lambda into two new constants \mu^2′ -> G\mu^2 and \lambda’ -> G\lambda. Except for the previous transformation, the terms containing the two constants are the same then in FST beacause they only depend on scalars.

iv) If in CST the gauge invariance of the Higgs field is the same as in FST the VEV has the same form as before and thus also v. In this case the contribution of the CST – namely the factor G – would cancel out and, therefore, all particles would obtain the same mass from the Higgs mechanism then in FST.

v) However, there are other parts in the Lagrangian which depend on vectorial quantities and, thus, transform not only by the factor G but also by multiplying with the metric or replacing partial derivatives by covariant derivatives. Because of this fact the solutions of the equations obtained from the Lagrangian in CST should be different from those in FST, which means the Higgs field (which is the solution) is different.

vi) A Lagrangian in CST should have a different gauge invariance then in FST and thus the field in CST also should have another gauge invariance then in FST. In this case probably the VEV will have a different form and then it could be that the contributions from the CST do not cancel out. In this case the particles would get a different mass in CST then in FST.

vii) The open questions are then: Do the Lagrangian has other symmetries in CST then in FST? How do they affect the VEV? And finally, what does this means for the mass the particles get through the Higgs mechanism.